今日も英語脳で英語を読む練習をしていきましょう!
一日一文“英語脳”で読む英語上達法
2023年1月14日(土)号
VOL.5030
本日の例文
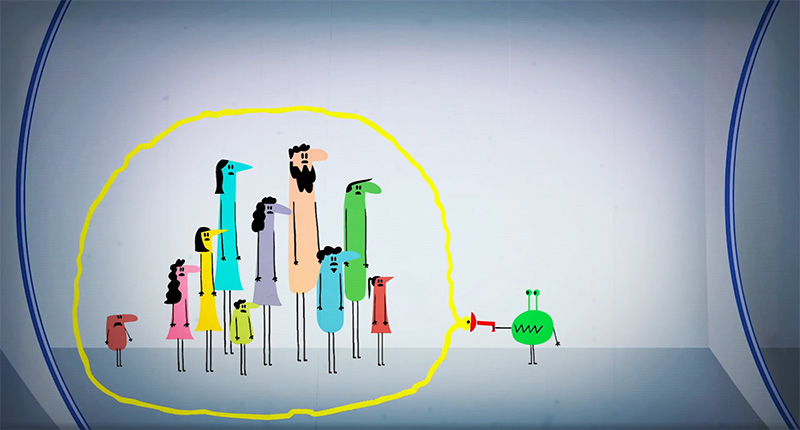
TED-Edより「Can you solve the prisoner hat riddle?(囚人の帽子クイズに挑戦)」です。
It can’t be the total number of black or white hats. There are more than two possible values, but what does have two possible values is that number’s parity, that is whether it’s odd or even. So the solution is to agree that whoever goes first will, for example, say “black” if he sees an odd number of black hats and “white” if he sees an even number of black hats.
今日の予習
■possible value
可能値
□parity
音声
[パ]・リ・ティ
(数学)偶奇性、パリティ
□solution
音声
ソ・[ル]ー・ション
問題解決策、解法
□whoever
音声
フー・[エ]ヴァー
~するのは誰でも
□deduce
音声
ディ・[デュ]ース
推定[推測・推論]する
■odd number
奇数
■even number
偶数
※
引用元:Can you solve the prisoner hat riddle? – TED
It can’t be the total number of black or white hats. There are more than two possible values, but what does have two possible values is that number’s parity, that is whether it’s odd or even. So the solution is to agree that whoever goes first will, for example, say “black” if he sees an odd number of black hats and “white” if he sees an even number of black hats.
で「(割り当てるべき意味は)白と黒の帽子それぞれの総数にするわけにいきません。それらは2つ以上の値になる可能性がありますので。でも、ぴったり2つの値にになるものと言えば、その数のパリティです。つまりその数が偶数か奇数かということです。ですから、解決方法は以下のことをみんなで事前に示し合わせることです。例えば、だれでもいいので最初になる人が、もし黒の帽子が奇数個あるのが見えたら『黒』と言い、もし偶数個あるのが見えたら『白』と言う。」という意味になります。
前回の文を確認したい方はこちらから。
http://www.eigonou.net/backnumber/05023/
It can’t be the total number of black or white hats. で「それは白と黒の帽子それぞれの総数にはなりえない」です。
it は what meaning can be assigned to those words「それらの言葉にどんな意味を割り当てられるか」を受けています。
There are more than two possible values, で「2個以上の値になる可能性がある」です。
だから、白と黒の帽子それぞれの総数を割り当てることはできない、というわけです。
but「しかし」
what does have two possible values is ~ で「まさに2個の値を持つものは~」です。
what does have two possible values で「ぴったり2個の値を持つもの」という意味でここでは主語になっています。
does have と強調の do を使って「ちょうど、ぴったり、まさに(~を持っている)」という意味を出しています。
ちょうど2個の値を持っているのは、
that number’s parity, で「その数のパリティ」です。
parity は数学用語で「偶奇性」です。
that is whether it’s odd or even. で「つまり、偶数か奇数かということです。」です。
So ~「だから」
the solution is to agree that ~ で「解決方法は、~ということに合意すること」です。
agree ~ で「~に合意する、同意する」です。つまりここではみんなで「示し合わせておく」ということです。
whoever goes first が主語で「初めに行く誰でも」です。
つまり「一番手」ですね。
for example,「例えば」
will say “black” で「黒と言う」です。
if he sees an odd number of black hats で「もし彼が~を見たら、奇数を、黒い帽子の」です。
he は先ほどの whoever を受けています。
an odd number of black hats で「黒い帽子が奇数個あること」です。
and「また」
(will say) “white” で「白と言う」です。
will say が省略されています。
if he sees an even number of black hats. で「もし彼が~を見たら、偶数を、黒い帽子の」です。
それではまず文節に分けて日本語に訳し内容を理解して下さい。
It can’t be the total number of black or white hats.
白と黒の帽子それぞれの総数にするわけにいきません。
There are more than two possible values,
2つ以上の値になる可能性があります
but
でも
what does have two possible values is
ぴったり2つの値になるものは~
that number’s parity,
その数のパリティです
that is
つまり
whether it’s odd or even.
奇数か偶数かです。
So
ですので
the solution is
その問題の解決方法は
to agree that
~ということを合意しておくこと
whoever goes first
誰でも一番手になる人が
will, for example, say “black”
例えば、黒と言います
if he sees an odd number of black hats
もし黒い帽子の数が奇数であるのが見えたら
and “white”
また、白と言います
if he sees an even number of black hats.
もし黒い帽子の数が偶数であるのが見えたら
今度は「頭の中で」一行ずつ日本語に訳しながら…
It can’t be the total number of black or white hats.
There are more than two possible values,
but
what does have two possible values is
that number’s parity,
that is
whether it’s odd or even.
So
the solution is
to agree that
whoever goes first
will, for example, say “black”
if he sees an odd number of black hats
and “white”
if he sees an even number of black hats.
次はいちいち日本語に訳さずに読んでみましょう。
It can’t be the total number of black or white hats.
There are more than two possible values,
but
what does have two possible values is
that number’s parity,
that is
whether it’s odd or even.
So
the solution is
to agree that
whoever goes first
will, for example, say “black”
if he sees an odd number of black hats
and “white”
if he sees an even number of black hats.
では最後に日本語に訳さずにこの文章の情景を思い浮かべられるようになるまで繰り返し読んでみてください。目を閉じて暗唱できるようになったら完璧です。
It can’t be the total number of black or white hats.
There are more than two possible values,
but
what does have two possible values is
that number’s parity,
that is
whether it’s odd or even.
So
the solution is
to agree that
whoever goes first
will, for example, say “black”
if he sees an odd number of black hats
and “white”
if he sees an even number of black hats.
Good job!
英語脳で読めましたね。今日も一歩、あなたはネイティブスピーカーに近づきました。おめでとう!
復習:日本語から英語を思い出してみましょう。
白と黒の帽子それぞれの総数にするわけにいきません。
2つ以上の値になる可能性があります
でも
ぴったり2つの値になるものは~
その数のパリティです
つまり
奇数か偶数かです。
ですので
その問題の解決方法は
~ということを合意しておくこと
誰でも一番手になる人が
例えば、黒と言います
もし黒い帽子の数が奇数であるのが見えたら
また、白と言います
もし黒い帽子の数が偶数であるのが見えたら
今日のつぶやき
今日の学習メモ
★多読:
You Are a Badass – by Jen Sincero(18%)
https://amzn.to/3mZCkLs
★スピーキング・リスニング:
Elevate – Brain Training
https://apps.apple.com/jp/app/elevate-brain-training/id875063456
英検1級過去問リスニング
★ボキャビル:
メルマガ発行
英検1級 文で覚える単熟語
https://amzn.to/34w6BtY
★その他:
初めて出会った言葉
aft「船尾の方へ」
fore-and-aft「船首から船尾まで」
毎日の継続が第一です。
それではまた明日!
メルマガ/フェイスブック/twitterでも毎日購読できます。
http://www.eigonou.net/mailmagazine/
http://www.facebook.com/eigonounet
https://twitter.com/eigonounet
是非ご参加ください!
みんなの英語学習法
http://www.eigonou.net/category/toukoumethod/
英語脳とは…
http://www.eigonou.net/
バックナンバー
http://www.eigonou.net/backnumber/
編集:こばやし
メルマガバックナンバー検索
全メルマガ記事5752件
The Japan Times Alpha(ジャパンタイムズアルファ) あらゆるレベルの人に購読をオススメする英字新聞です








コメントを残す